![\includegraphics[width=13.5cm]{bondwire}](img2415.png)
|
Wire inductors, so called bond wire connections, are used to connect active and passive circuit components as well as micro devices to the real world.
The freespace inductance ![]() of a wire of diameter
of a wire of diameter ![]() and length
and length ![]() is given [46,47] by
is given [46,47] by
![$\displaystyle L = \dfrac{\mu_0}{2\pi} \cdot l \left[ \ln\left\{ \frac{2l}{d} + ...
...)^2} \right\} + \frac{d}{2l} - \sqrt{1+\left(\frac{d}{2l}\right)^2} + C \right]$](img2417.png) |
(11.235) |
where the frequency-dependent correction factor ![]() is a function of
bond wire diameter and its material skin depth
is a function of
bond wire diameter and its material skin depth ![]() is expressed
as
is expressed
as
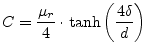 |
(11.236) |
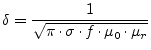 |
(11.237) |
where ![]() is the conductivity of the wire material. When
is the conductivity of the wire material. When
![]() is small,
is small,
![]() . The wire resistance
. The wire resistance ![]() is given
by
is given
by
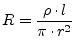 |
(11.238) |
with
![]() and
and ![]() .
.
The effect of the ground plane on the inductance valueof a wire has
also been considered. If the wire is at a distance ![]() above the
ground plane, it sees its image at
above the
ground plane, it sees its image at ![]() from it. The wire and its
image result in a mutual inductance. Since the image wire carries a
current opposite to the current flow in the bond wire, the effective
inductance of the bond wire becomes
from it. The wire and its
image result in a mutual inductance. Since the image wire carries a
current opposite to the current flow in the bond wire, the effective
inductance of the bond wire becomes
![$\displaystyle L = \dfrac{\mu_0}{2\pi}\cdot l \left[ \ln\left( \dfrac{4h}{d} \ri...
...^2}{l^2}} - \sqrt{1+\dfrac{d^2}{4l^2}} - 2 \dfrac{h}{l} + \dfrac{d}{2l} \right]$](img2427.png) |
(11.239) |
Mirror is a strange model that is frequency independent. Whereas computations are valid, hypothesis are arguable. Indeed, they did the assumption that the ground plane is perfect that is really a zero order model in the high frequency domain.